Conduct and Interpret a One-Sample T-Test
What is the One-Sample T-Test?
The one-sample t-test is a member of the t-test family. All the tests in the t-test family compare differences in mean scores of continuous-level (interval or ratio), normally distributed data. Unlike the independent or dependent-sample t-tests, the one-sample t-test works with only one mean score. The one-sample t-test compares the mean of a single sample to a predetermined value to determine if the sample mean is significantly greater or less than that value.
The independent sample t-test compares the mean of one distinct group to the mean of another group. An example research question for an independent sample t-test would be, “Do boys and girls differ in their SAT scores?” The dependent sample t-test compares two matched scores or measurements (such as before vs. after). An example research question for a dependent sample t-test would be, “Do pupils’ grades improve after they receive tutoring?”
On the other hand, the one-sample t-test compares the mean score found in an observed sample to some predetermined or hypothetical value. Typically, the hypothetical value is the population mean or some other theoretically derived value.
Some possible applications of the one-sample t-test include testing a sample against a predetermined or expected value, testing a sample against a certain benchmark, or testing the results of a replicated experiment against the original study. For example, a researcher may want to determine if the average age of retiring in a certain population is 65. The researcher would draw a representative sample of people entering retirement and ask at what age they retired. A one-sample t-test could then be conducted to compare the mean age obtained in the sample (e.g., 63) to the hypothetical test value of 65. The t-test determines whether the difference we find in our sample is larger than we would expect to see by chance.
The One-Sample T-Test in SPSS
In this example, we will conduct a one-sample t-test to determine if the average age of a population of students is significantly greater or less than 9.5 years.
Need help with your analysis?
Schedule a time to speak with an expert using the calendar below.
User-friendly Software
Transform raw data into written, interpreted, APA formatted t-test results in seconds.
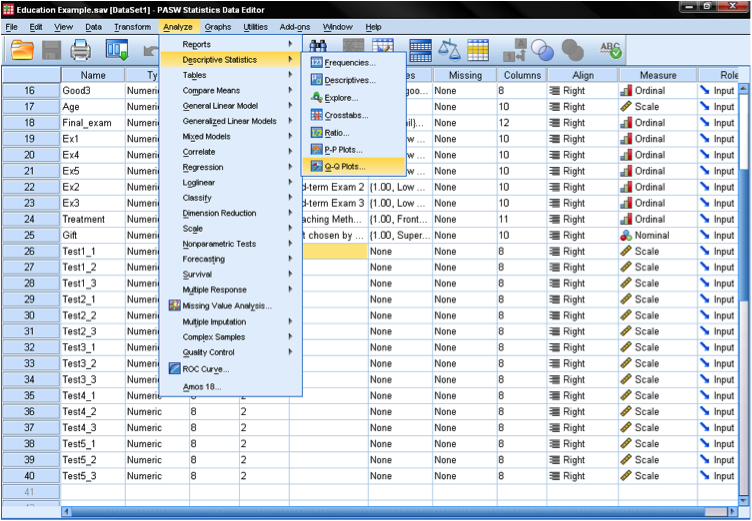
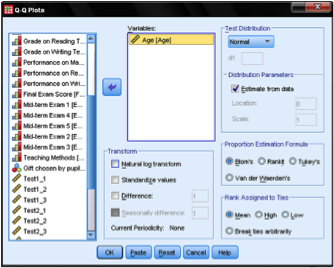
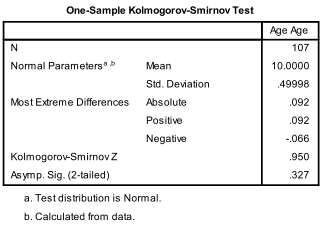
Before we actually conduct the one-sample t-test, our first step is to check the distribution for normality. This may be done with a Q-Q Plot (located under Analyze > Descriptive Statistics in SPSS). Then we simply add the variable we want to test (age) to the box and confirm that the test distribution is set to Normal. This will create the diagram you see below. The output shows that small values and large values somewhat deviate from normality. As an additional check, we can run a Kolmogorov-Smirnov (K-S) test to test the null hypothesis that the variable is normally distributed. We find here that the K-S test is not significant; thus, we cannot reject the null hypothesis and may assume that age is normally distributed.
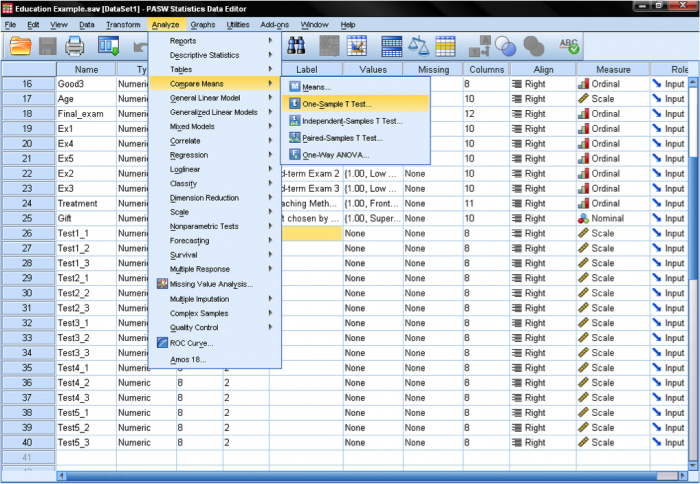
Let’s move on to the one-sample t-test, which can be found in Analyze > Compare Means > One-Sample T Test…
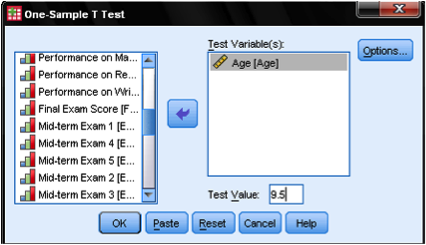
The one-sample t-test dialog box is fairly simple. We add the test variable age to the list of Test Variables and then enter the Test Value. In our case, the hypothetical test value is 9.5. The dialog Options… gives us the setting for how to manage missing values and also the opportunity to specify the width of the confidence interval used for testing.
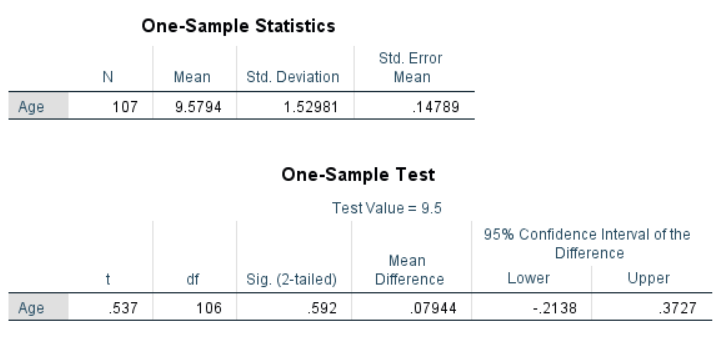
Once all of the appropriate options are set, click OK to run the analysis. The figure below shows the output. The “One-Sample Statistics” section shows descriptive statistics for the sample, including the mean being compared to the test value. The “One-Sample Test” section shows the results of the t-test. In this case, the null hypothesis is that the mean of the sample is equal to 9.5. For the purpose of this example, we will set our significance (alpha) level to .05. The Sig. column displays the p-value for the test. The results show that the p-value (.592) is greater than .05. This suggests that the null hypothesis cannot be rejected, and the age of the sample is not significantly different from 9.5.