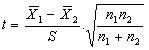Independent Sample T-Test
Independent samples t-test is a statistical technique that is used to analyze the mean comparison of two independent groups. When we take two samples from the same population, then the mean of the two samples may be identical. But when samples are taken from two different populations, then the mean of the sample may differ. In this case, it is used to draw conclusions about the means of two populations, and used to tell whether or not they are similar.
Assumptions:
1. Assumes that the dependent variable is normally distributed.
2. Assumes that the variance of the two groups are the same as the dependent variable.
3. Assumes that the two samples are independent of each other.
4. Samples are drawn from the population at random.
5. All observations must be independent of each other.
6. Dependent variables must be measured on an interval or ratio scale.
Procedure:
1. Set up the hypothesis.
a. Null Hypothesis: It is assumed when the means of the two groups are not significantly different.
b. Alternative Hypothesis: Assumes that the means of the two groups are significantly different.
2. Calculate the standard deviation by using this formula:
Need help with your analysis?
Schedule a time to speak with an expert using the calendar below.
User Friendly Software
Turn raw data into written, interpreted, APA formatted t-test results in seconds.
V= degree of freedom
N1+N2= number of observations in both samples.
5. Hypothesis testing: Statistical decisions are made about whether or not the two population means are identical. Compare the calculated value of the independent sample t-test with the table value. If the calculated value is greater than the table value of the predetermined significance level, we will reject the null hypothesis and say that the means of the two groups are different. If the calculated value of the is less than the table value, then we will say that the means of the two groups are the same.
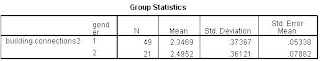
SPSS:
2. Click on the “open data” icon and select the data.
3. Click on the “analysis” option and select “compare mean” from the analysis option.
4. Select “independent sample t-test” from the compare mean option, the following window will appear: